Hello ICTM members! My name is Angie Shindelar and I serve on the ICTM Board as the Vice-President for Elementary. I previously taught elementary and middle school math at Nodaway Valley CSD. I am currently a Math Consultant for Green Hills AEA.
My recent articles have discussed effective instruction for addition and subtraction basic facts. You can find the articles on the ICTM website in the three previous newsletters if you are interested and missed them. In this article I continue with the basic fact theme but turn the focus to thinking about the learning progression prior to basic fact instruction. Understanding this progression and being intentional about building a foundation for basic fact instruction can ensure our students’ success in achieving fluency for addition and subtraction basic facts in a timely way. These critical understandings are important for K-2 teachers but also for teachers working with older students that are struggling to make sense of basic fact strategies fluency.
Prior to instruction in basic facts students should develop understanding of parts and wholes. There are three critical components to this work:
1) subitizing
2) knowing plus 1 and minus 1
3) composing and decomposing numbers
Below is a description of each component and a list of Iowa Core Math Standards that encompass the learning for each component.
The first critical component of developing understanding of parts and wholes is subitizing. Subitizing can be described as instantly seeing how many without the need to count. The human brain can instantly tell “how many” of up to 5 objects without having to count. Beyond 5, our brains have to break a quantity into smaller chunks. Subitizing experiences provide students opportunities to think about quantities in different arrangements and using different models. A typical subitizing experience is when a teacher quickly flashes a quantity for students to see and ask students, “How many do you see?” The teacher asks several students to tell how many they saw and how they knew how many there were. Students will describe everything from attempts to count the items individually, relating to arrangements they are familiar with, and describing smaller parts within the whole.
The quantity might be represented with a dot arrangement, a five/ten frame, or a rekenrek (number rack). Below are examples of different arrangements of a quantity. To build understanding of parts and wholes, students should experience visualizing quantities in various arrangements and with the different models.
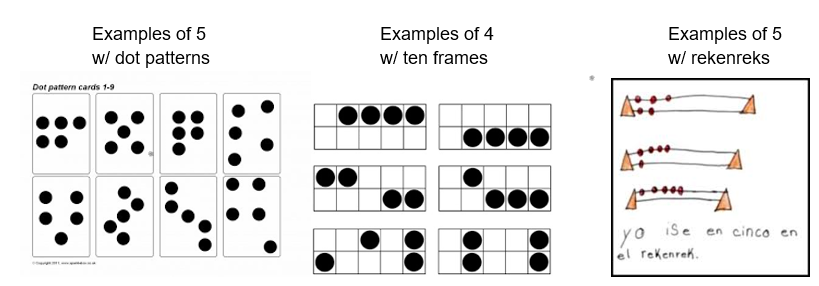
When subitizing is a regular routine, students first become familiar with arrangements up to 5. Once the arrangements move beyond 5 the goal shifts to looking for the parts within the whole. Students learn to resist the temptation to count and instead focus on the arrangement looking for the smaller parts. For example, when shown the quantity of 6 in the arrangement below, students may agree there is 6 but describe how they knew differently. Some may see 3 and 3, while others noticed 4 and 2. By working on subitizing as a regular routine, students learn to look for parts they recognize in quantities typically up to 10.

One of the best resources for subitizing is Number Talks by Sherry Parrish. There is an entire section on subitizing with many examples of arrangements and models for quantities up to 10. This book really helped me understand the importance of providing different arrangements for each number and varying the model.
While subitizing is not specifically referred to in the Iowa Core Math Standards, it lives within the Counting and Cardinality standards. You can find references to subitizing when you read further about the standards and unpack the specific learning within them. This standard relates most closely to the subitizing work:
Understand the relationship between numbers and quantities; connect counting to cardinality. (K.CC.4.)
The second critical component of developing understanding of parts and wholes is plus 1 and minus 1. This can be tricky, because we often think of it as facts like 5 + 1 or 4 -1. However, prior to working on basic facts in this format, it is critical to build understanding of what plus 1 and minus 1 actually mean. Students in early grades spend a considerable amount of time counting and thinking about what number comes next, what is one more than, what number comes before, and what is one less than. They develop this understanding by connecting it to the count sequence and are typically successful and confident. However, when given problems like 5 + 1 or 4 - 1, too often we see students not make the connection of what is one more than, what number comes before, and what is one less than to addition and subtraction. It is often baffling to teachers as they see students counting fingers to solve.
As students are learning about these important understandings, it is critical to make explicit the connections to the language of addition and subtraction and representing with equations.
Explicitly discussing and modeling that one more than and what number comes next is written as +1 is critical to students making connections. They have to see visual models, hear the language relationship to addition and subtraction, and have opportunities to use mathematical symbols by writing equations to represent the plus 1 and minus 1 concepts.
Word problems also help students make connections to addition and subtraction. Posing addition and subtraction word problems as a regular part of students’ learning provides opportunities to use the language of one more or one less and make connections to addition and subtraction. Including connections to equations is key to this work. Here are examples of intentionally connecting the concept of plus 1 and minus 1 in word problems:
Sam has 6 toy cars. His brother gave him 1 more. How many toy cars does Sam have now?
Mei has 7 cookies. Rob has 1 less cookie than Mei. How many cookies does Rob have?
Students work on finding ways to represent the problem and solve. While sharing ways to represent, the teacher brings forward the discussion of what 1 more or 1 less means and how to record mathematically with an equation.
While plus 1 and minus 1 is not specifically referred to in the Iowa Core Math Standards, it lives within both the Counting and Cardinality and the Operations and Algebraic standards. You can find references when you read further about the standards and unpack the specific learning within them. The standards that encompass plus 1 and minus 1 are listed here:
-
Count forward beginning from a given number within the known sequence instead of having to begin at 1. (K.CC.2.)
-
Understand the relationship between numbers and quantities; connect counting to cardinality. (K.CC.4.)
-
Represent addition and subtraction with objects, fingers, mental images, drawings, sounds (e.g., claps), acting out situations, verbal explanations, expressions, or equations. (K.OA.1.)
-
Solve addition and subtraction word problems, and add and subtract within 10, e.g., by using objects or drawings to represent the problem. (K.OA.2.)
-
Fluently add and subtract within 5. (K.OA.5.)
The third critical component of developing understanding of parts and wholes is composing and decomposing numbers. Understanding that any number can be composed and decomposed in different ways is essential to later fact fluency work. For example, think about all the ways to make 6. Through exploring ways to make 6, for example, students build understanding of the quantity 6 as well as patterns and properties. Discussions of the combinations can highlight patterns like when we have 3 and 3, we can take away 1 from one group of 3 and give it to the other group of 3. So now have 2 and 4. If we again take away 1 from the group of 2 and give it to the group of 4, we now have 1 and 5, etc. Or we might highlight that 4 and 2 is a way to make 6, and we also see that 2 and 4 are shown. Exploring this brings forward discussion of the commutative property for addition.
Providing regular opportunities for students to engage with composing and decomposing numbers is essential. Discussion of students’ findings and connections to patterns and properties will deepen understanding of parts and wholes. Resources that highlight activities for parts and wholes understanding are Student-Centered Mathematics by John Van de Walle and Developing Number Concepts, Book 2 by Kathy Richardson. Here are a few activities I have assembled in this document based upon activities I have used from these resources.
In addition, the Put Together Take Apart problem type that requires finding both addends is a perfect opportunity for students to work on composing and decomposing. Below are examples:
Composing and decomposing numbers is specifically referred to in the Iowa Core Math Standards in the Operations and Algebraic standards. You can find more specific references when you read further about the standards and unpack the specific learning within them. The standards that encompass composing and decomposing numbers are listed here:
-
Represent addition and subtraction with objects, fingers, mental images, drawings, sounds (e.g., claps), acting out situations, verbal explanations, expressions, or equations. (K.OA.1.)
-
Solve addition and subtraction word problems, and add and subtract within 10, e.g., by using objects or drawings to represent the problem. (K.OA.2.)
-
Decompose numbers less than or equal to 10 into pairs in more than one way, e.g., by using objects or drawings, and record each decomposition by a drawing or equation (e.g., 5 = 2 + 3 and 5 = 4 + 1). (K.OA.3.)
-
For any number from 1 to 9, find the number that makes 10 when added to the given number, e.g., by using objects or drawings, and record the answer with a drawing or equation. (K.OA.4.)
-
Fluently add and subtract within 5. (K.OA.5.)
Note: There are first grade standards that also could be included, however I chose not to include them because the intent is to describe essential learning prior to working on basic fact strategies.
These three critical components for developing understanding of parts and wholes set students up for success as they begin formal work in fact strategies. Students that are learning fact strategies with ease and steadily working toward achieving fluency have a solid understanding of parts and wholes. Contrastly, when students are struggling with learning fact strategies and are not progressing as expected toward achieving fluency, you can almost guarantee the gap in their learning centers around unfinished learning around parts and wholes.
I am always interested in your thoughts and feedback for any of the topics I discuss in the ICTM newsletter. You can reach me at ashindelar@ghaea.org.
